Hi everyone. Well this is something I feel is what an engineer should know as for our mathematics is a very important tool. But most of the times people feel its sort off forced upon them as necessary thing to sort off rattofy and omit. Our schools only concentrated upon the calculation part. But what Arthur Benjamin feels is Maths is made up of three parts:
1. Calculation
2. Application
3. Inspiration
Yes I highlighted the third part because this is what almost all schools or colleges fail upon...inspiration. Can Maths be inspirational? Boy you are kidding me... But hey this post will highlight certain key points, which, I really hope will help you motivate to find that inspiration behind mathematics.
To start with I will talk on Fibonacci series.
So what is Fibonacci series? To give a short explanation, its a series of infinite numbers wherein each element is made up by sum of previous two elements. So the number series looks like the following:
0 1 1 2 3 5 8 13 21 34 55 89 144 233 377....
This sequence of numbers was introduced to the western world by an Italian mathematician Leonardo Pisano Bigollo also known as Fibonacci in 1202 AD. And by the way, to all Indians out there , the thing is that this sequence was known to Indians way back before Fibonacci, but as he introduced it to the western world ,it was named after him. (Thats how it goes folks...anyways).
So whats so special about this sequence? Well one thing is one can square all the numbers and if you are a good observant you should see a pattern..
0 1 1 4 9 25 64 169 441 1156 3025 ...
Now add the numbers..what do you see?
0+1=1
1+1=2
1+4=5
4+9=13
9+25=34
25+64=89
And that my dear friends is seen as a pattern in Fibonacci series as shown:
0 1 1 2 3 5 8 13 21 34 55 89 144 233 377...
So ya this was interesting isn't it?
If you still don't find anything amusing then check this out.
1+1+4=6
1+1+4+9=15
1+1+4+9+25=40
1+1+4+9+25+64=104
OK SO? Whats so special ? You only added the squares..
Special thing is:
1+1+4=6=2*3
1+1+4+9=15=3*5
1+1+4+9+25=40=5*8
1+1+4+9+25+64=104=8*13
which is...Fibonacci again!!!
Now lets try and look why this is happening.
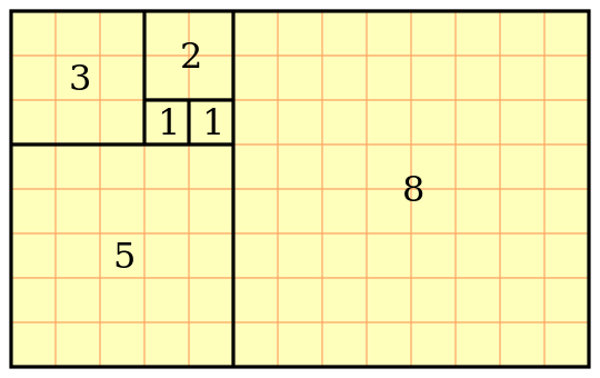
Here I took squares of number (as literally a square as area of square is length*length).
So what is area of this big rectangle? Add area of individual squares and that becomes
1+1+4+9+25+64=104
And area of rectangle is length*breadth= 8*13=104.
So yeah pretty simple.
I am gonna go ahead and talk about another thing which I think if you don't know yet, then maybe, it will blow your mind...!!!!!!
Now divide the numbers as follows:
Lets start with 13 and 8
13/8 =1.625
21/13 =1.615
34/21 =1.619
55/34= 1.6176
and so on...
This result converges to a number 1.6180. So whats so special about this number? Well to those who don't know, this is known as the "Golden Ratio".
Ok so?
Ya I know what's so mind blowing about it. What if I tell you that you knew number right from your birth even if you read about it just now.... Don't believe me? Continue to read more for I am about to tell you something special..
If I connect the previous diagram number 1, it may look something like this:

So does this look familiar? This is what we call in simple terms spiral. And I think now you are starting to know what I was intending to say so far..
Coming to what Benjamin said the second part of mathematics is application.
So heres some real life application of same:
1) Taj Mahal:
Yea folks our own wonder of world - Taj Mahal is based on Golden Ratio. See the pic and believe it:

Doesn't this look like our first diagram?
2) Greek Architecture:

3) Honey Bees:

1. Calculation
2. Application
3. Inspiration
Yes I highlighted the third part because this is what almost all schools or colleges fail upon...inspiration. Can Maths be inspirational? Boy you are kidding me... But hey this post will highlight certain key points, which, I really hope will help you motivate to find that inspiration behind mathematics.
To start with I will talk on Fibonacci series.
So what is Fibonacci series? To give a short explanation, its a series of infinite numbers wherein each element is made up by sum of previous two elements. So the number series looks like the following:
0 1 1 2 3 5 8 13 21 34 55 89 144 233 377....
This sequence of numbers was introduced to the western world by an Italian mathematician Leonardo Pisano Bigollo also known as Fibonacci in 1202 AD. And by the way, to all Indians out there , the thing is that this sequence was known to Indians way back before Fibonacci, but as he introduced it to the western world ,it was named after him. (Thats how it goes folks...anyways).
So whats so special about this sequence? Well one thing is one can square all the numbers and if you are a good observant you should see a pattern..
0 1 1 4 9 25 64 169 441 1156 3025 ...
Now add the numbers..what do you see?
0+1=1
1+1=2
1+4=5
4+9=13
9+25=34
25+64=89
And that my dear friends is seen as a pattern in Fibonacci series as shown:
0 1 1 2 3 5 8 13 21 34 55 89 144 233 377...
So ya this was interesting isn't it?
If you still don't find anything amusing then check this out.
1+1+4=6
1+1+4+9=15
1+1+4+9+25=40
1+1+4+9+25+64=104
OK SO? Whats so special ? You only added the squares..
Special thing is:
1+1+4=6=2*3
1+1+4+9=15=3*5
1+1+4+9+25=40=5*8
1+1+4+9+25+64=104=8*13
which is...Fibonacci again!!!
Now lets try and look why this is happening.

Here I took squares of number (as literally a square as area of square is length*length).
So what is area of this big rectangle? Add area of individual squares and that becomes
1+1+4+9+25+64=104
And area of rectangle is length*breadth= 8*13=104.
So yeah pretty simple.
I am gonna go ahead and talk about another thing which I think if you don't know yet, then maybe, it will blow your mind...!!!!!!
Now divide the numbers as follows:
Lets start with 13 and 8
13/8 =1.625
21/13 =1.615
34/21 =1.619
55/34= 1.6176
and so on...
This result converges to a number 1.6180. So whats so special about this number? Well to those who don't know, this is known as the "Golden Ratio".
Ok so?
Ya I know what's so mind blowing about it. What if I tell you that you knew number right from your birth even if you read about it just now.... Don't believe me? Continue to read more for I am about to tell you something special..
If I connect the previous diagram number 1, it may look something like this:

So does this look familiar? This is what we call in simple terms spiral. And I think now you are starting to know what I was intending to say so far..
Coming to what Benjamin said the second part of mathematics is application.
So heres some real life application of same:
1) Taj Mahal:
Yea folks our own wonder of world - Taj Mahal is based on Golden Ratio. See the pic and believe it:

Doesn't this look like our first diagram?
2) Greek Architecture:
3) Honey Bees:

Both the Fibonacci numbers and the Golden Ratio appear in Honeybees.
The Fibonacci numbers are very well represented in honeybees. For example, the if you follow the family tree of honeybees, it follows the fibonacci sequence perfectly. If you taken any hive and follow this pattern, it would look like this:
If you divide the number of female bees by the number of males bees you get 1.618, the golden ratio. This mathematical sequence work for any honeybee hive at any give time. Commonly, honeybee hives are always used to explain the Fibonacci Sequence and Golden Ratio
And many other things like petals, shell of a snail, Human DNA (yes really, the double helix structure follows golden ratio), the galaxy itself is made of Golden Ratio....
Even our face structure is made up of golden ratio(not everyone's ..we only tend to... but those who have face in this ratio are most handsome in the world.... Yea George Clooney I know...)
Its like God must be a great Engineer (I don't mind calling him that) to be able to create the perhaps complex looking world relatively simpler on mere application of a single ratio. (Hence engineer is a perfect word as most of us often make simple answers look complicated in our exams as well😜 )
So thats it folk..... Oh btw was I able to bring in some sort of Inspiration for you in maths.. write about it in comments section..
Till then... Happy mathing folks... (I don't know why I said that..)

Comments
Post a Comment